MAXIMA AND MINIMA OF FUNCTIONS

TO FIND THE MAXIMA AND MINIMA OF FUNCTIONS FOLLOWING STEPS ARE FOLLOW:

• Let the given function is Y=0.
• Now differentiate the function w.r.t. to X.
• Differentiate of Y w.r.t. X is slope of curve.
• Now minimum and maximum, put slope of curve is zero.
• From above condition we get value of X.
• Now double differentiate the Y.
• Now put the value of X in Double differentiate.
• If double differentiate is less than zero then maxima will be occur at that point.
• If double differentiate is greater than zero then minima will be occur at that point.
• If double differentiate is  zero then that point is called saddle point.

          

For example:

Let f(x)= y = x³ − 6x² + 12x − 5

now differentiate w.r.t. to X.
that is
y = 3x² − 12x + 12 
For minima and maxima put Y=0
that is 3x² − 12x + 12=0

we get X=2 only

Now double differentiate of f(x)
y'' = 6x − 12
 put X=2 in the double derivative we get
y''=6*2-12=0
hence the test is fail and at X=2, the curve has neither has maxima and minima. This point is called saddle point.

EXAMPLE:
 Find the maxima and minima for:
y = 5x³ + 2x² − 3x

Now first differentiate and find the slope of curve.
i.e.

y’=15x²+4x-3
put slope is equal to zero i.e. 15x²+4x-3=0
we get

x = −3/5 and x = +1/3

Now double differentiate the function we get

y″=30x+4

Now at x = −3/5 value of y″=30(-3/5)+4=(-14)

and

at x = +1/3

y″=30(1/3)+4=14

Hence y″<0 at x = −3/5 and y″>0

from this we can conclude that the function has maxima at  x = −3/5 minima at x = +1/3

THE GRAPH OF FUNCTION IS SHOWN BELOW:

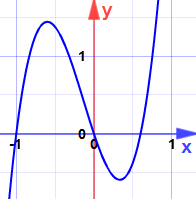
Determine the maxima and minima of the function y=x³-x²-x+1.

First find the slope of the function

y′=3x²-2x-1

slope is zero when 3x²-2x-1=0

when

3x²-2x-1=0

3x²-3x+x-1=0

3x(x-1)+1(x-1)=0

(3x+1)(x-1)=0

from this x=(-1/3) and x=1

find double differentiation i.e. y″

y″=6x-2

y″(-1/3)=6×(-1/3)-2

y″(-1/3)=-4

and x=1

y″(1)=6×1-2=4

y″(1)=4

y″(1)>0 hence function has minima at x=1.

and

y″(-1/3)<0 hence function has maxima at x=-1/3

Minimum value

y(1)=1³-1²-1+1=0

and

Maximum value

y(-1/3)=(-1/3)³-(-1/3)²-(-1/3)+1=1.185

y(-1/3)=1.185